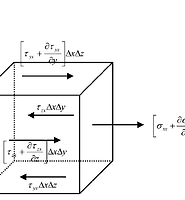
대학원 공부노트
Hasegawa(1969) 5번 식 해설 본문
이번 페이지에서 다룰 내용은 Fourier-Legendre series를 다룰 예정입니다.
목표는 아래 두 식을 유도해내는 것이에요.
Step 1: Legendre Polynomial
To derive above equations, we need 'Legendre polynomial'
Similarily, we can express
And coefficient
(We derived/studied 'orthogonality' before.)
According to orthogonality under \(m\neq n)
So, equation (1) will be...
Step 2: Rodrigues formula
We derived/studied 'Rodrigues formula' before.
Let's put this equation in our integral form.
As it is too complex to solve at once, use integration by parts.
Reference
Advanced Engineering Mathematics p.178 SEC 5.2 Legendre's equation. Legendre polynomials
'대학원 공부 > Hasegawa' 카테고리의 다른 글
| Hasegawa equation (0) | 2022.08.05 |
|---|---|
| Hasegawa(1969) 1번 식 해설 4편(완) (0) | 2022.07.31 |
| Hasegawa(1969) 1번 식 해설 3편 (0) | 2022.07.29 |
| Hasegawa(1969) 1번 식 해설 2편 (0) | 2022.07.26 |
| Hasegawa(1969) 1번 식 해설 1편 (0) | 2022.07.25 |
Comments